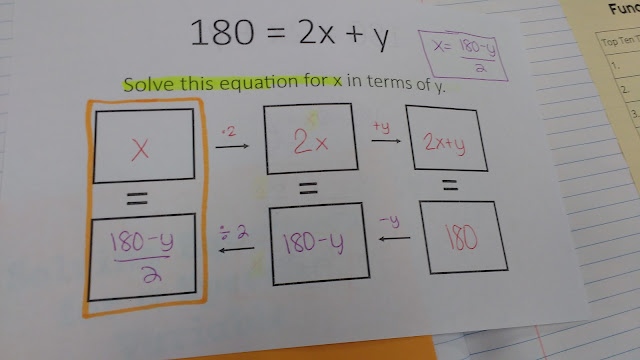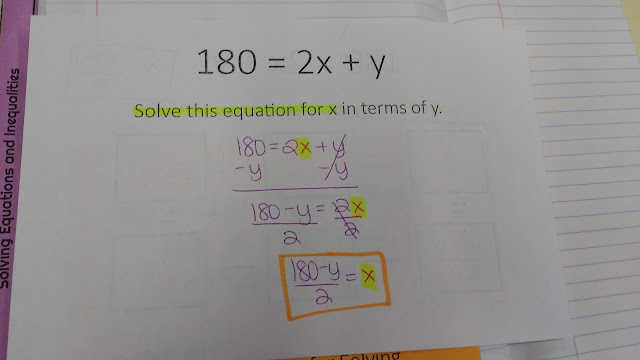We are currently working on relations and functions in Algebra 1 which is by far my favorite unit of the year. This means I can finally share our solving equations and inequalities unit with the internet!
This is my second year teaching Algebra 1 under the new Oklahoma Academic Standards. And, I'll be honest; I'm still trying to wrap my mind around what exactly the standards entail and how best to go about teaching them. This year's unit on solving equations went 100 times better than last year. Having said that, I still have so many changes I would like to implement in the future. I'm proud of what we did this year, but I know that I can still do more. Next year, I want to implement more application problems.
Every unit starts out with a divider. One side of the divider has a place for students to list the "Top Ten Things to Remember" from this unit. At the end of each unit, students get a grade for completing this as part of their notebook checks. Some students keep a running list as we go through the unit. Other students wait until right before the notebook check to write their list. Still others leave it blank. I can't win every battle.
The opposite side of the divider has places for students to record their quiz scores for each skill.
You can find out more about these dividers and download a template here.
Before beginning to solve equations and inequalities, we did a quick review of inverse operations.
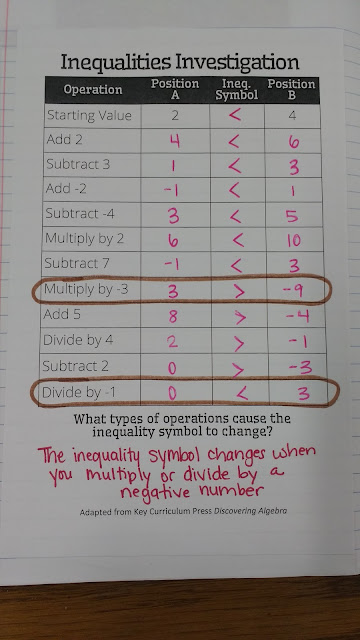
We followed this up with an inequalities investigation that was inspired by an activity from Discovering Algebra (affiliate link) called "Toe the Line." The goal of this activity is to remind students that we need to flip the inequality symbol whenever we multiply or divide both sides of the inequality by a negative number.
Up Next: A summary of steps for solving equations/inequalities with variables on one side.
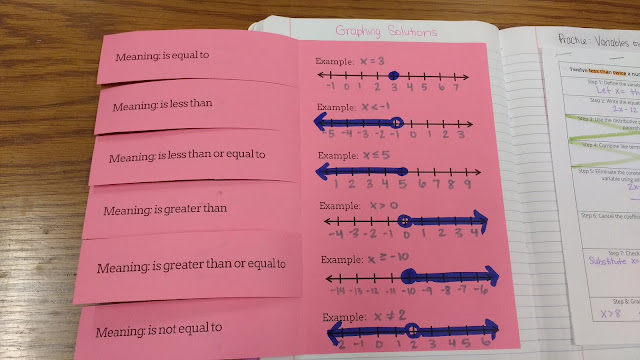
Next, we did a quick review of how to graph solutions on a number line. For my students, this is all a review except for graphing "not equals to."
In the past, I did this without the equal sign. I'm SO thankful I finally found time to add the equal sign this year.
We did six practice problems in our notebook over solving equations/inequalities with variables on one side. Students stapled these problems together and glued them on a single page. Some students even chose to staple their paper with the steps on top of the practice problems to condense things even further. I kinda like this approach!
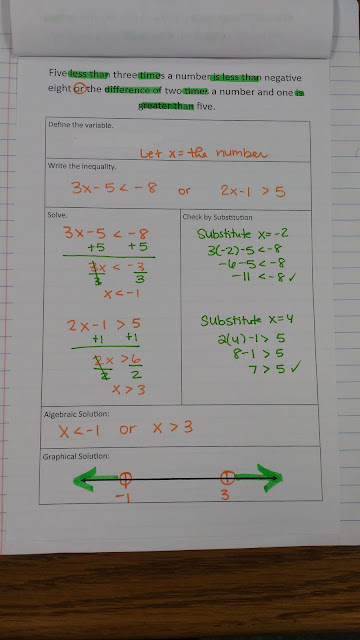
At the end of our first unit, students were really struggling with translating between words and algebra. So, I decided to continue giving my students practice translating by giving them every single equation and inequality in WORDS instead of ALGEBRA. Was this the right decision? I'm not really sure. But, I can say that half way through this unit on solving equations/inequalities, my students started translating between words and algebra like bosses! The continued practice did end up paying off. What it prevented, however, was the chance to look at different types of application problems. I would like to combine these two approaches when I teach this again.
Here are close-ups of each:
Next time, I do want to make a few changes to this template. I want to switch around steps 7 and 8. By having my students check their solutions before they graphed them, some of my students became confused and started putting the results they got from checking their work on the number line instead of the solution they found.
To introduce the idea of solving equations with variables on both sides, I gave them a set of scale puzzles that I downloaded from Sarah Rubin at Everybody is a Genius. I love giving these puzzles to my students and giving them 3 minutes to solve them. There are only two rules:
1. Both sides of the scale must balance.
2. Whatever number is placed in one box on the scale must be placed in every box on that scale.
Students have a hard time following rule #2. Of course, the reason they want to break rule #2 is that the some of the problems they are trying to solve are actually impossible.
Our next foldable was also inspired by Sarah Rubin.
As a twist, I had my students create their own equations.
A New Set of Steps: Solving Equations/Inequalities with Variables on BOTH Sides
Practice Problems:
Here are close-ups of each:
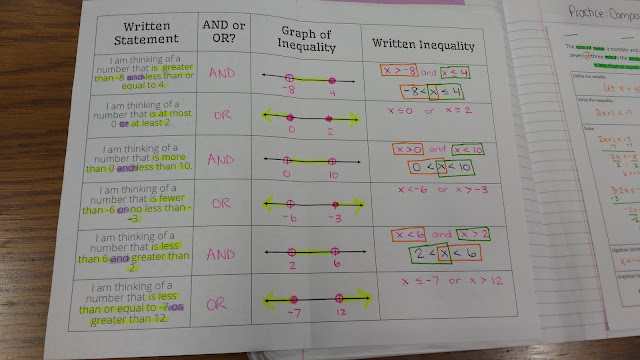
I reused a foldable I made last year for compound inequalities. I'm still not happy with how well my students understand the differences between "AND" inequalities and "OR" inequalities. I need to rethink my approach to this in the future.
We only did four practice problems in our notebooks for compound inequalities. I think we should have done more.
Here are close-ups of each:
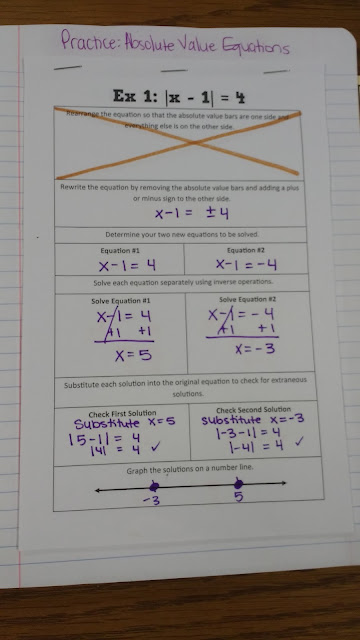
Steps for Solving Absolute Value Equations
Practice problems are next.
Close-ups of each problem are below.
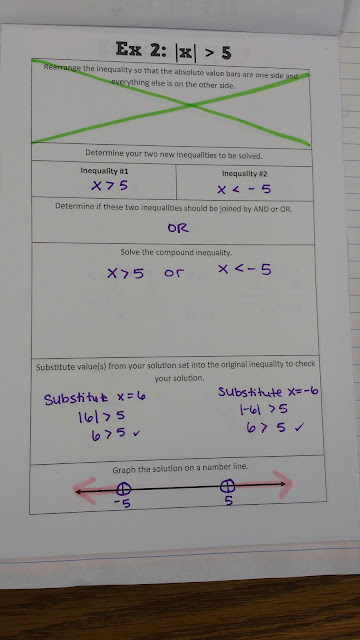
Steps for Solving Absolute Value Inequalities
Practice Problems:
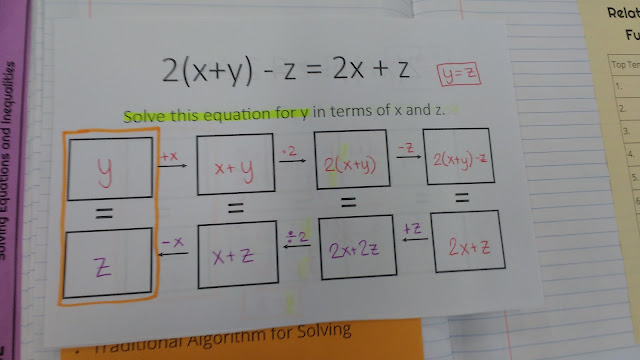
Our last skill for the unit is solving equations for a specific variable. This is also known as solving literal equations or changing the subject of the equation.
I let my students choose between two methods of solving: flowchart method and the more traditional algorithm for solving equations.
We did four practice problems twice each.
You can download the files for this unit here.
This is my second year teaching Algebra 1 under the new Oklahoma Academic Standards. And, I'll be honest; I'm still trying to wrap my mind around what exactly the standards entail and how best to go about teaching them. This year's unit on solving equations went 100 times better than last year. Having said that, I still have so many changes I would like to implement in the future. I'm proud of what we did this year, but I know that I can still do more. Next year, I want to implement more application problems.
Every unit starts out with a divider. One side of the divider has a place for students to list the "Top Ten Things to Remember" from this unit. At the end of each unit, students get a grade for completing this as part of their notebook checks. Some students keep a running list as we go through the unit. Other students wait until right before the notebook check to write their list. Still others leave it blank. I can't win every battle.
The opposite side of the divider has places for students to record their quiz scores for each skill.
You can find out more about these dividers and download a template here.
Before beginning to solve equations and inequalities, we did a quick review of inverse operations.
We followed this up with an inequalities investigation that was inspired by an activity from Discovering Algebra (affiliate link) called "Toe the Line." The goal of this activity is to remind students that we need to flip the inequality symbol whenever we multiply or divide both sides of the inequality by a negative number.
Up Next: A summary of steps for solving equations/inequalities with variables on one side.
Next, we did a quick review of how to graph solutions on a number line. For my students, this is all a review except for graphing "not equals to."
In the past, I did this without the equal sign. I'm SO thankful I finally found time to add the equal sign this year.
We did six practice problems in our notebook over solving equations/inequalities with variables on one side. Students stapled these problems together and glued them on a single page. Some students even chose to staple their paper with the steps on top of the practice problems to condense things even further. I kinda like this approach!
At the end of our first unit, students were really struggling with translating between words and algebra. So, I decided to continue giving my students practice translating by giving them every single equation and inequality in WORDS instead of ALGEBRA. Was this the right decision? I'm not really sure. But, I can say that half way through this unit on solving equations/inequalities, my students started translating between words and algebra like bosses! The continued practice did end up paying off. What it prevented, however, was the chance to look at different types of application problems. I would like to combine these two approaches when I teach this again.
Here are close-ups of each:
Next time, I do want to make a few changes to this template. I want to switch around steps 7 and 8. By having my students check their solutions before they graphed them, some of my students became confused and started putting the results they got from checking their work on the number line instead of the solution they found.
To introduce the idea of solving equations with variables on both sides, I gave them a set of scale puzzles that I downloaded from Sarah Rubin at Everybody is a Genius. I love giving these puzzles to my students and giving them 3 minutes to solve them. There are only two rules:
1. Both sides of the scale must balance.
2. Whatever number is placed in one box on the scale must be placed in every box on that scale.
Students have a hard time following rule #2. Of course, the reason they want to break rule #2 is that the some of the problems they are trying to solve are actually impossible.
Our next foldable was also inspired by Sarah Rubin.
As a twist, I had my students create their own equations.
A New Set of Steps: Solving Equations/Inequalities with Variables on BOTH Sides
Practice Problems:
Here are close-ups of each:
I reused a foldable I made last year for compound inequalities. I'm still not happy with how well my students understand the differences between "AND" inequalities and "OR" inequalities. I need to rethink my approach to this in the future.
We only did four practice problems in our notebooks for compound inequalities. I think we should have done more.
Here are close-ups of each:
Steps for Solving Absolute Value Equations
Practice problems are next.
Close-ups of each problem are below.
Steps for Solving Absolute Value Inequalities
Practice Problems:
Our last skill for the unit is solving equations for a specific variable. This is also known as solving literal equations or changing the subject of the equation.
I let my students choose between two methods of solving: flowchart method and the more traditional algorithm for solving equations.
We did four practice problems twice each.
You can download the files for this unit here.