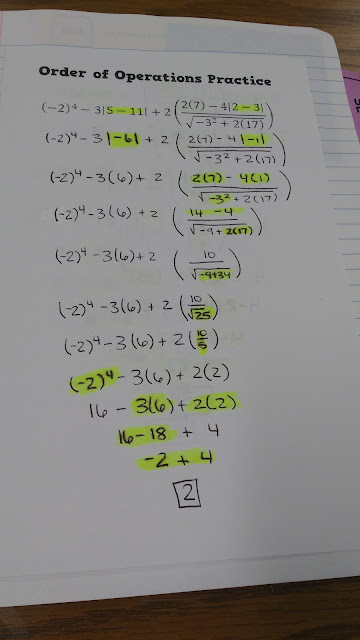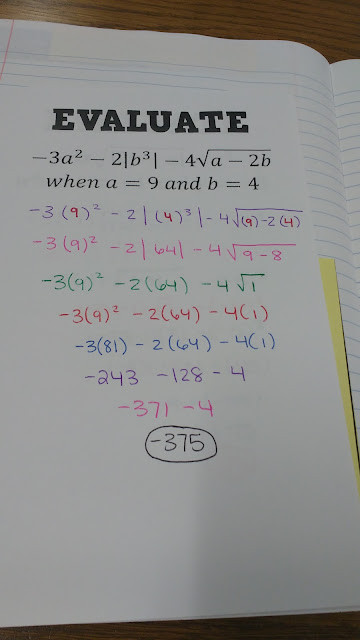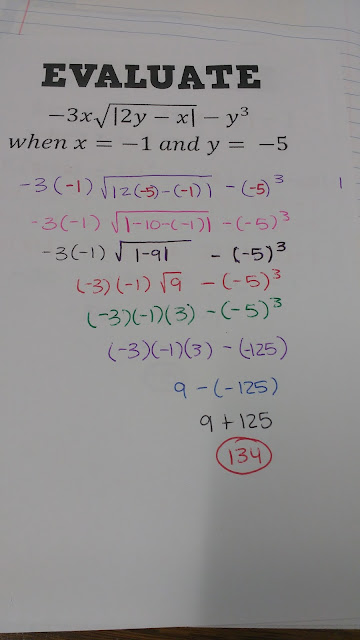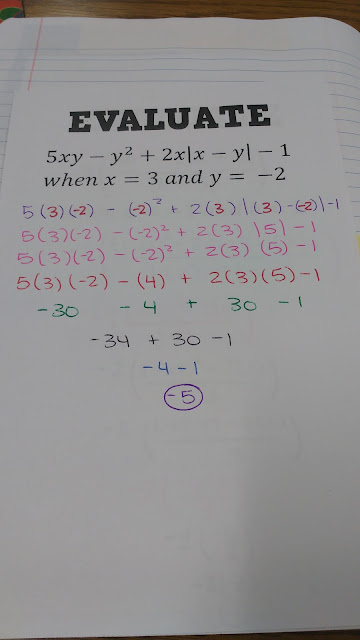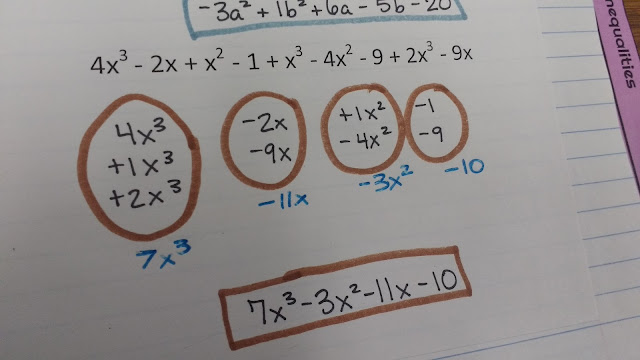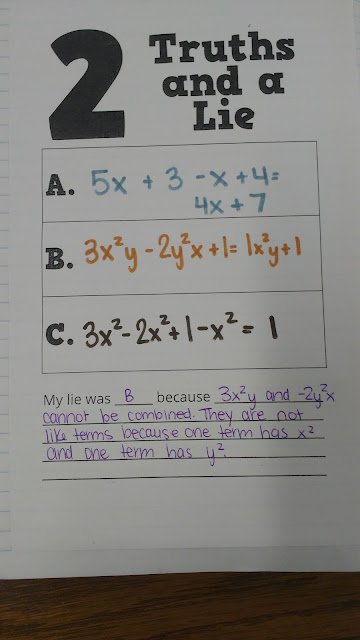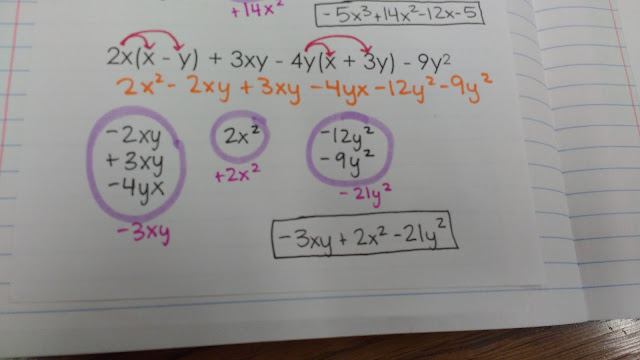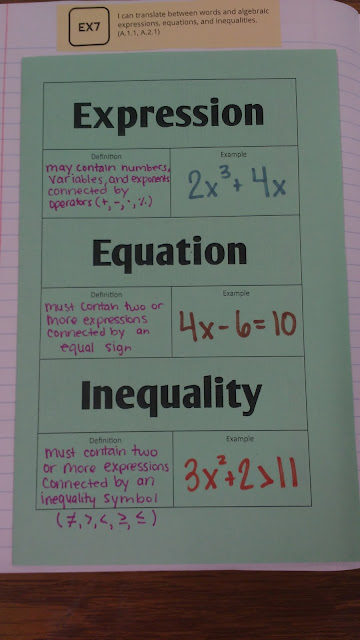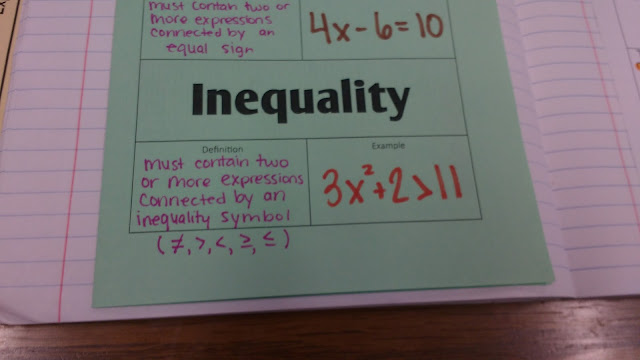Unit 1 in Algebra 1 was a long one. And, it took us much longer than I expected to get through it. Now that we're out of our introductory unit on expressions, I think we're finally making some headway through Algebra 1. And, I hope to get caught back up to where we should be by Christmas. We have had quite a few disruptions this year, and it has definitely showed in our pacing.
In this post, I want to share pictures of each of our interactive notebook pages from the first unit. I have already blogged about some of these pages in individual blog posts, but many are being shared for the first time in this post.
Every unit starts with a divider. These dividers have really taken my interactive notebooks to the next level. My students' notebooks are ten times more organized now than before I started using dividers between each unit. Learn more about these dividers here.
Our first skill of the unit was over four key words: absolute value, reciprocal, opposite, and opposite reciprocal. We completed a frayer model for each word. This year, I am pre-printing the words on the frayer models. In years past, I had so many students forget to write the word in the center. That's the most important part!
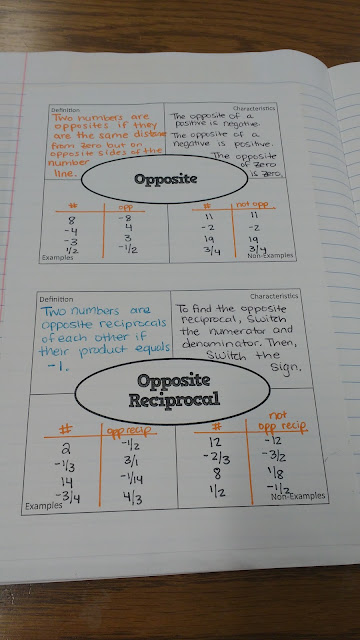
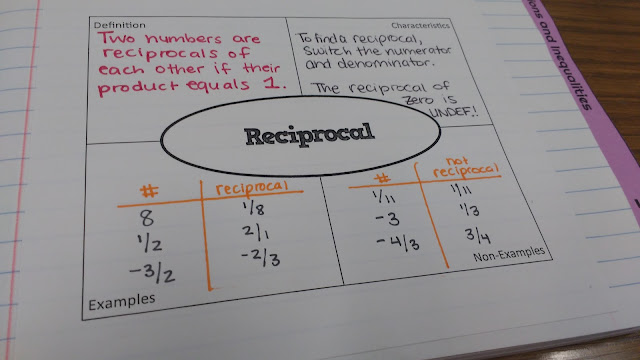
Reciprocal:
Opposite Reciprocal:
We completed an Always, Sometimes, Never task involving these vocabulary words. I blogged more about this activity here.
We also did a 2 Truths and a Lie activity where students had to apply their knowledge of our new vocabulary words. This 2 Truths and a Lie template can be used for almost any topic. You can download it here.
Our second skill of the unit was a review of the order of operations.
We began with an examination of the connections between negatives, exponents, and parentheses. Next year, I think I'll make this it's own skill.
I downsized the grouping symbols poster from my wall so students could have a version in their notebooks.
We also put in a diagram of the order of operations. I made it to look like the order of operations posters on my wall.
We did three practice order of operations problems in our notebooks.
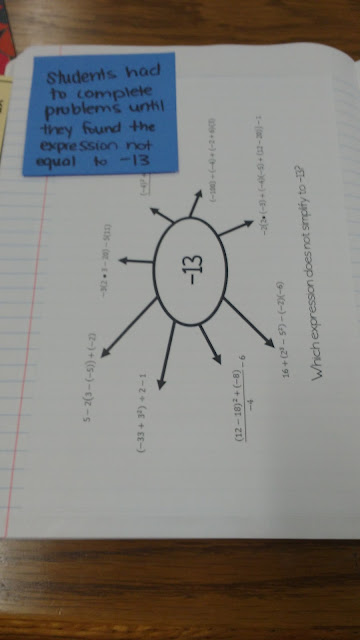
To give students extra practice, I gave them a puzzle created by Greta Bergman.
Students had to evaluate expressions until they found the one that didn't equal -13.
Once we were pretty comfortable with solving order of operations problems, it was time to begin evaluating algebraic expressions.
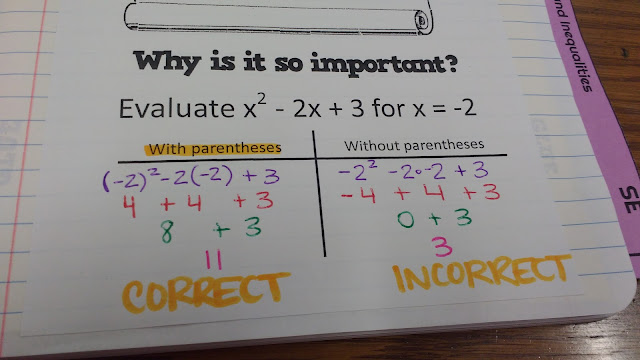
Every year I have my students sign what I now call the "Parenthetical Promise." They promise to always, always, always use parentheses with substituting values into an algebraic expression.
This year, I decided to take it a step farther and illustrate to students what happens when we substitute without parentheses. I had them verify the evaluation on their calculators using the store feature to show that the method with parentheses actually is correct.
We created an interactive notebook pocket to hold our evaluating algebraic expressions practice problems.
The steps for evaluating expressions was printed on the pocket.
Here were our practice problems:
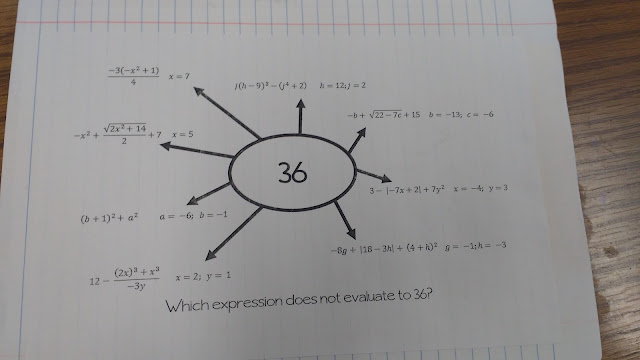
For extra practice, we did another "One Incorrect" puzzle from Greta Bergman. Students could stop evaluating expressions once they had proven to me they had found that one that didn't evaluate to 36.
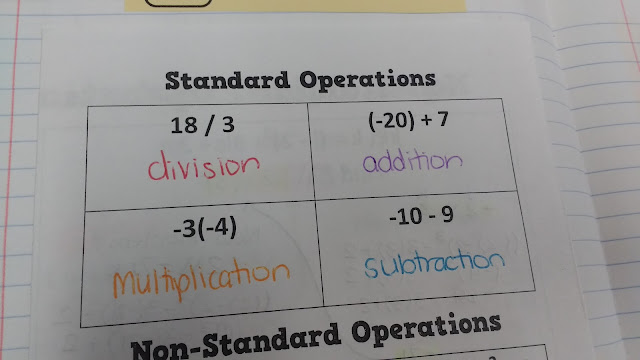
The next skill was Non-Standard Operations. This is new to the Oklahoma standards, and this is only the second year that I have ever had to teach it. Last year, I tried adding non-standard operations into the same skill as evaluating algebraic expressions. It did NOT go well. This year, it got it's own skill, and things went MUCH better.
First, we did a quick review of standard operations to help us wrap our minds around non-standard operations.
Then, we tackled one-step non-standard operations problems.
Just as my students were starting to get the hang of one-step non-standard operation problems, I threw them in the deep end with multi-step problems.
We did two two-step problems and two three-step problems.
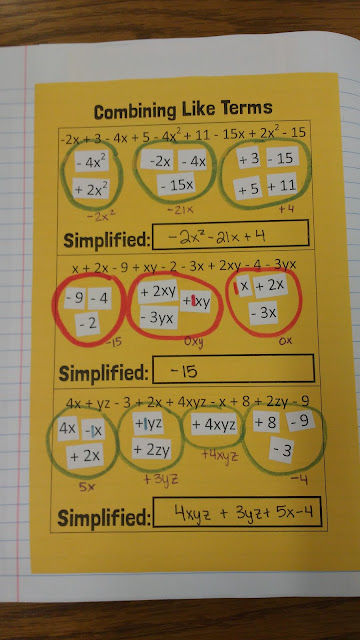
Next up, combining like terms! We began with a "Like Terms" Frayer Model.
I modified my combining like terms strip activity that I have used for the past two years. This year, I added a box for students to write their simplified answer in. I think it really helped them organize their work better!
Close-ups of each problem:
We still needed a bit more practice. Plus, there's the fact that we can't always cut our terms apart and glue them into groups! Sometimes we just have to use pencil and paper, so we need to learn how.
My students really took to the idea of placing them in distinct groups of like terms this year.
We followed up this practice sheet with another 2 Truths and a Lie activity.
Now, on to the distributive property!
First, we tackled a super simple problem that's like what my students were used to seeing from middle school.
Then, a slightly harder problem that involves drawing in the invisible 1 in front of the parentheses.
Then, LOTS of practice inside!
Those practice problems were pretty easy, so I made another practice sheet with questions similar to what would be on their quiz. After taking the quiz, one student asked me if I meant to make the questions on this page look like the questions on the quiz. Why, yes, I did!
Close-ups of each problem:
And, lucky us, Greta Bergman has also made a "One Incorrect" puzzle for distributive property!
Now, it's time for three new vocab words: expression, equation, and inequality.
We wrote definitions for each and created examples as a class.
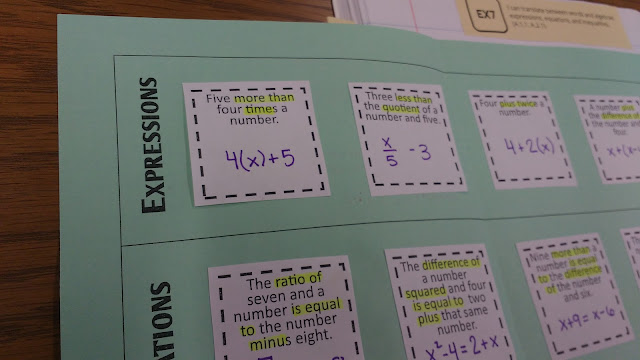
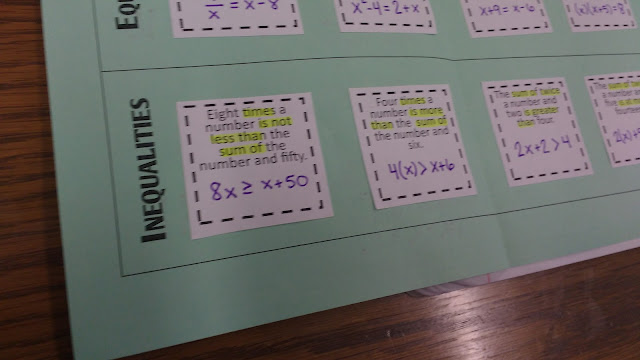
Up next, more practice with translating. Some of my students really got into the make your own problem section!
I've made myself a note to change the wording on one of the problems because it's a bit ambiguous and could be written two different ways.
I decided to reuse an expression writing practice sheet that I made to use with my students two years ago. We no longer take an end-of-instruction exam that features these types of problems, but it did give my students a bit of extra practice with translating from words to symbols!
And, that's all our notes for the first unit of the year in Algebra 1.
We did also glue in two things to our reference materials section at the front of our notebooks.
First, we glued in a translating "cheat sheet" that I created last year.
Students tri-fold it before gluing it in so that it can unfold and be used no matter where we are in our notebook.
When we aren't using it, it folds up nicely in our notebook.
If students glue it on the left hand page of their notebook, it can be used throughout the rest of the year!
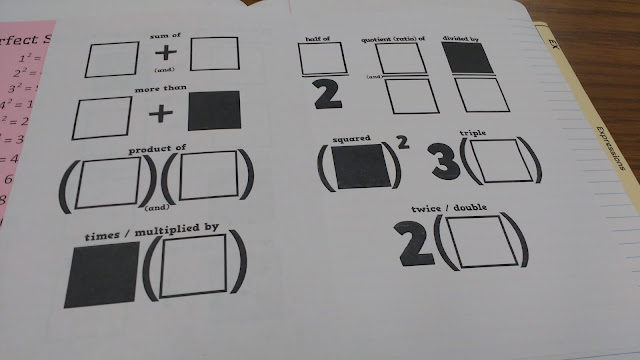
The other reference we glued in also had to do with translating from words to algebra. I created a set of translating cards as an experiment to see if it helped students with their translation. I'd link to the blog post sharing the cards, but I haven't got around to blogging about them yet.
After using the cards, my students expressed a wish to have a copy of the cards for their notebooks. I'm not entirely happy with this notebook version, but the whole system needs some tweaks before I use it again. Still, my students have found it to be a useful resource in their notebooks!
You can download the files for this unit here.
In this post, I want to share pictures of each of our interactive notebook pages from the first unit. I have already blogged about some of these pages in individual blog posts, but many are being shared for the first time in this post.
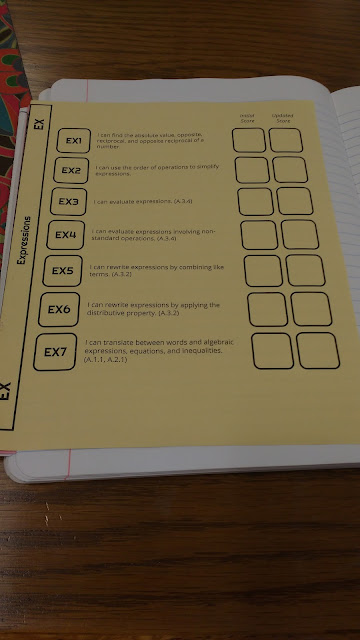
Every unit starts with a divider. These dividers have really taken my interactive notebooks to the next level. My students' notebooks are ten times more organized now than before I started using dividers between each unit. Learn more about these dividers here.
The left side of the divider asks students to list the top ten things they think they need to remember from this unit. Some of my students wait until the end of the unit to fill this out. Others fill it in as we go. And, to be honest, some never fill it out at all. These students do, however, lose points for this when I do their notebook check at the end of the unit. For more information about how I'm currently doing notebook checks, read this post.
The right side of the divider lists our SBG skills for the unit. Students are expected to record their quiz scores in their notebook whenever quizzes are handed back out. I also check this when I do notebook checks.
Our first skill of the unit was over four key words: absolute value, reciprocal, opposite, and opposite reciprocal. We completed a frayer model for each word. This year, I am pre-printing the words on the frayer models. In years past, I had so many students forget to write the word in the center. That's the most important part!
Here are close-ups of each Frayer model.
Absolute Value:
Reciprocal:
Opposite:
Opposite Reciprocal:
We completed an Always, Sometimes, Never task involving these vocabulary words. I blogged more about this activity here.
We also did a 2 Truths and a Lie activity where students had to apply their knowledge of our new vocabulary words. This 2 Truths and a Lie template can be used for almost any topic. You can download it here.
Our second skill of the unit was a review of the order of operations.
We began with an examination of the connections between negatives, exponents, and parentheses. Next year, I think I'll make this it's own skill.
I downsized the grouping symbols poster from my wall so students could have a version in their notebooks.
We also put in a diagram of the order of operations. I made it to look like the order of operations posters on my wall.
We did three practice order of operations problems in our notebooks.
To give students extra practice, I gave them a puzzle created by Greta Bergman.
Students had to evaluate expressions until they found the one that didn't equal -13.
Once we were pretty comfortable with solving order of operations problems, it was time to begin evaluating algebraic expressions.
Every year I have my students sign what I now call the "Parenthetical Promise." They promise to always, always, always use parentheses with substituting values into an algebraic expression.
This year, I decided to take it a step farther and illustrate to students what happens when we substitute without parentheses. I had them verify the evaluation on their calculators using the store feature to show that the method with parentheses actually is correct.
We created an interactive notebook pocket to hold our evaluating algebraic expressions practice problems.
The steps for evaluating expressions was printed on the pocket.
Here were our practice problems:
For extra practice, we did another "One Incorrect" puzzle from Greta Bergman. Students could stop evaluating expressions once they had proven to me they had found that one that didn't evaluate to 36.
The next skill was Non-Standard Operations. This is new to the Oklahoma standards, and this is only the second year that I have ever had to teach it. Last year, I tried adding non-standard operations into the same skill as evaluating algebraic expressions. It did NOT go well. This year, it got it's own skill, and things went MUCH better.
First, we did a quick review of standard operations to help us wrap our minds around non-standard operations.
Then, we tackled one-step non-standard operations problems.
Just as my students were starting to get the hang of one-step non-standard operation problems, I threw them in the deep end with multi-step problems.
We did two two-step problems and two three-step problems.
Next up, combining like terms! We began with a "Like Terms" Frayer Model.
I modified my combining like terms strip activity that I have used for the past two years. This year, I added a box for students to write their simplified answer in. I think it really helped them organize their work better!
Close-ups of each problem:
We still needed a bit more practice. Plus, there's the fact that we can't always cut our terms apart and glue them into groups! Sometimes we just have to use pencil and paper, so we need to learn how.
My students really took to the idea of placing them in distinct groups of like terms this year.
We followed up this practice sheet with another 2 Truths and a Lie activity.
Now, on to the distributive property!
First, we tackled a super simple problem that's like what my students were used to seeing from middle school.
Then, a slightly harder problem that involves drawing in the invisible 1 in front of the parentheses.
Then, LOTS of practice inside!
Those practice problems were pretty easy, so I made another practice sheet with questions similar to what would be on their quiz. After taking the quiz, one student asked me if I meant to make the questions on this page look like the questions on the quiz. Why, yes, I did!
Close-ups of each problem:
And, lucky us, Greta Bergman has also made a "One Incorrect" puzzle for distributive property!
Now, it's time for three new vocab words: expression, equation, and inequality.
We wrote definitions for each and created examples as a class.
On the inside, students translated twelve statements written in words to symbols. Then, they classified each statement as an expression, equation, or inequality.
Up next, more practice with translating. Some of my students really got into the make your own problem section!
I've made myself a note to change the wording on one of the problems because it's a bit ambiguous and could be written two different ways.
I decided to reuse an expression writing practice sheet that I made to use with my students two years ago. We no longer take an end-of-instruction exam that features these types of problems, but it did give my students a bit of extra practice with translating from words to symbols!
And, that's all our notes for the first unit of the year in Algebra 1.
We did also glue in two things to our reference materials section at the front of our notebooks.
First, we glued in a translating "cheat sheet" that I created last year.
Students tri-fold it before gluing it in so that it can unfold and be used no matter where we are in our notebook.
When we aren't using it, it folds up nicely in our notebook.
If students glue it on the left hand page of their notebook, it can be used throughout the rest of the year!
The other reference we glued in also had to do with translating from words to algebra. I created a set of translating cards as an experiment to see if it helped students with their translation. I'd link to the blog post sharing the cards, but I haven't got around to blogging about them yet.
After using the cards, my students expressed a wish to have a copy of the cards for their notebooks. I'm not entirely happy with this notebook version, but the whole system needs some tweaks before I use it again. Still, my students have found it to be a useful resource in their notebooks!
You can download the files for this unit here.